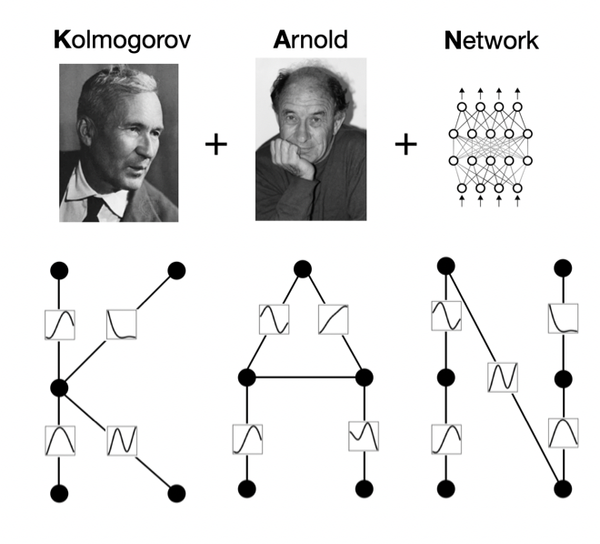
A Cantor set is a set of points obtained by successive deletion of open middle thirds from a closed interval. It is named after the German mathematician Georg Cantor. The Cantor set is an example of a self-similar set, meaning that it is a set whose components can be divided into smaller copies of the whole set.
To create the Cantor set, start with the unit interval [0,1]. Remove the open middle third (1/3,2/3). This leaves four segments: [0, 1/3], [1/3, 2/3], [2/3, 1]. Now remove the middle third of each of these remaining segments, leaving eight segments. Continue this process of deleting the middle third of each segment indefinitely. The Cantor set is the closure of the resulting set of points.

The Cantor set is a perfect example of a zero-dimensional set, meaning that it has no measurement of length, area, or volume. It is also uncountable, meaning that it is infinite, but not in a "countable" way. The Cantor set has a measure of zero, meaning that it has no length, area, or volume. Intuitively, this means that if you were to draw the Cantor set on a piece of paper, it would take up no space.
The Cantor set has many interesting properties. It is totally disconnected, meaning that it is composed of disjoint closed sets. This means that there are no two points in the set which are connected. The Cantor set is also nowhere dense, meaning that it has no interior points. This means that there are no points in the Cantor set which can be "filled in" with more points. Finally, the Cantor set is perfect, meaning that it is equal to its own boundary.
The Cantor set is an important example in mathematics and is used to illustrate many concepts in set theory, topology, and measure theory. It is also used to prove the Cantor-Bernstein-Schroeder theorem, which states that if two sets have the same cardinality, then they must be equivalent. This theorem is important in the study of countable sets.
Cantor-Bernstein-Schroeder theorem
The Cantor-Bernstein-Schroeder (CBS) theorem is a fundamental result in set theory. It states that if there exists a one-to-one correspondence between two sets A and B, then there exists a bijection (a one-to-one and onto function) between the two sets. This theorem is important in many areas of mathematics, including topology, real analysis, and group theory.
In set theory, a bijection is a function that maps each element in one set to exactly one element in another set. That is, for any two elements a and b in A, there is a unique element c in B such that f(a) = c and f(b) = c. This means that the function is both one-to-one and onto, or bijective.
The CBS theorem can be used to prove the existence of bijections between two sets. To prove the theorem, we first assume that there is a one-to-one correspondence between A and B. A one-to-one correspondence, or injection, is a function f from A to B such that for any two distinct elements a and b in A, f(a) does not equal f(b). We then construct a function g from B to A such that for any two distinct elements c and d in B, g(c) does not equal g(d). This shows that f and g are both injective functions and so, by the CBS theorem, there exists a bijection between A and B.
The CBS theorem is useful in many areas of mathematics. In topology, it can be used to prove the existence of homeomorphisms between two topological spaces. In real analysis, it is used to prove the existence of continuous functions between two spaces. In group theory, it can be used to prove the existence of homomorphisms between two groups.
The CBS theorem is an important result in set theory and is used in many areas of mathematics. It allows us to prove the existence of bijections between two sets and is used to prove the existence of many other mathematical objects.
Do you like our work?
Consider becoming a paying subscriber to support us!